

The ~ expresses the sequence of reactants, products, reaction intermediates and transition states and is usually undefined or only vaguely defined by the reaction coordinate (extent of bond breaking or bond making).
DEFINITION ABSCISSA SERIES
If we mark on an axis of ~e a series of lengths proportional to angles, and trace ordinates which are proportional to sines or other trigonometrical functions, we get periodic curves of a harmonic character. In the very simplest elementary reactions it might correspond to the str etching or twisting of a particular bond, and be shown to a scale. The " reaction coordinate" plotted along the abscissa represents the changes in atomic coordinates as the system progresses from reactants to products. Each of the points on the plane can be represented by numbers.įinally, a spatial coordinate system It nucleates three lines that are perpendicular to each other (called X, Y and Z), which meet at a point of origin (0) and whose points in space can be represented by three numbers.Latent variable versus observable variable The center of coordinates O corresponds to the value 0.Ī plane coordinate system, for its part, is composed of two perpendicular lines that intersect at their origin. Within the framework of a linear coordinate systemAny point that is part of a certain line can be linked and symbolized by means of a real number (which will be positive if it is a point located to the right of O or negative if it is in the left portion). DiscardsAs many of you will know, he is often regarded as the father of analytic geometry. In a plane, the Cartesian coordinate X is called abscissa, while the Cartesian coordinate Y is distinguished with the expression "Orderly".Įxperts in the field say that the Cartesian system has been named in honor of the philosopher, scientist and mathematician Rene Descartes ( 1596- 1650), who sought to support his philosophical reasoning from a starting point on which to build all knowledge. The system reference relative to an axis (a straight), two (one flat) or three axes (in the space) that are perpendicular to each other and that coincide at a certain point that is identified with the name of coordinates origin, is known as Cartesian coordinates. It was in the year 1801 that this work was published, which is written in Latin, where it enters fully into what is the fundamental theorem of algebra. In the same way, we must not overlook the structure that he gave to Number Theory and the large number of publications he made, among which he stands out Disquisitiones arithmeticae. This last author has gone down in the history of Mathematics for various issues and among them it should be noted, without a doubt, the fact that he was the first scientist to carry out what is the proof of the fundamental theorem of algebra. However, we cannot ignore that, in one way or another, it also "drinks from the waters" of differential geometry, developed by the German mathematician Carl Friedrich Gauss, and of algebraic geometry.
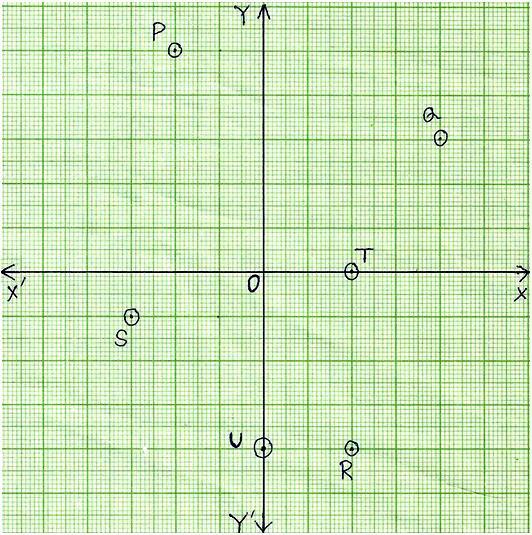
This area must be emphasized that it has its origin in Cartesian geometry, the movement that René Descartes would develop in the period between the seventeenth and eighteenth centuries. This is a scientific area that is responsible for carrying out what is the study of the various geometric figures through the use of a series of techniques, algebra and mathematical analysis, in what is a coordinate system. This term that concerns us and many others, such as equations or axes, are all fundamental and key concepts in what is called analytical geometry. The so-called abscissa axis represents the horizontal coordinate axis. It is known as abscissa (word derived from Latin abscissa, "cut") to a horizontal direction coordinate appearing on a rectangular Cartesian plane and that is expressed as the distance that exists between a point and the vertical axis.


 0 kommentar(er)
0 kommentar(er)
